Number Of Decks In Blackjack
- Blackjack Number Chart
- Number Of Decks In Blackjack Tournaments
- Number Of Decks In Blackjack Card Game
- Number Of Decks In Casino Blackjack
| 8 votes (61.53%) | |
| 1 vote (7.69%) | |
| 1 vote (7.69%) | |
| 2 votes (15.38%) | |
| No votes (0%) | |
| No votes (0%) | |
| No votes (0%) | |
| No votes (0%) | |
| No votes (0%) | |
| 3 votes (23.07%) |
Of the number of decks used, but decreasing probabilities of receiving a third card to remain in the game. Thus, casinos are more likely to administer games with more decks in play. The goal is to determine whether to hit' or stand' when dealt certain a pair. Keywords: 21, Blackjack, cards, combinatorial, combinatorics, probability. So the probability of drawing a blackjack from two decks is 4.78%. That translates as the greater the number of decks, the less probability of drawing a blackjack. In 6 decks the probability drops down to 4.75%. So a larger number of decks means slightly fewer blackjacks. In six decks, the probability drops further yet to 4.75%.
- The table shows that the impact of reduced blackjack odds is much greater than increasing the number of decks. So whilst you may hear “always play single deck” as advice, it is crucial to ensure that the other key rules are also the same. Playing a single deck game with a reduced 6/5 blackjack payout has a house edge roughly two and a half times that of an eight-deck game with the normal.
- How many decks are used in blackjack at a casinoThe game can be played with 1, 2, 4, 6, and 8 dec. How many decks are used in blackjack at a casino.
13 members have voted
 Wizard
WizardAdministrator
This post shall address my initial analysis, which I open up for peer review.
First, let's establish some baseline rules:
- Dealer hits soft 17
- Blackjack pays 3 to 2
- Dealer peeks for blackjack
- Player may double after a split
- Player may NOT surrender
- Player may re-split up to four hands, including aces
- Continuous shuffler used
- Total-dependent basic strategy followed
My house edge calculator shows the house edge as follows:
- One deck = 0.014%
- Eight decks = 0.577%
This makes the effect of the difference in number of decks 0.563%.
Before I go on, all figures in this analysis are the result of billions of hands played by random simulation.
Let's work on this like an onion, starting with a simplistic balanced version of blackjack and then add the effects of the rules that are not equal both ways.
Imagine a blackjack game where the player follows a 'mimic the dealer' strategy of hitting to 17 and also hitting a soft 17. Also let a winning blackjack pays 1-1. Finally, add a rule that if both the player and dealer bust, that the result is a push. This game is perfectly balanced for a zero house edge.
Next, let's remove the rule about a push if both player and dealer bust. Instead, we use the real blackjack rule that if both the player and dealer bust, then the dealer wins. Here is the player expected value adding that rule for one and eight decks:
- One deck = -8.237%
- Eight decks = -8.157%
- Difference = -0.079%.
Why does the player lose more with a single deck? It's because there is more busting on both sides in a single-deck game, resulting in more double busts. The absolute value of the percentages above, to be exact. Why is there more busting with a single deck? I figure it's because both sides are hitting hands with more small cards than large, resulting in hitting into a deck/shoe that is high-card rich. This effect of removal is simply stronger with a single deck.
Next, let's consider the value of a blackjack paying 3-2. It is easy to calculate the effect of this mathematically, as follows:
| Decks | Prob player BJ | Prob dealer BJ | Prob winning BJ | Value |
|---|---|---|---|---|
| 1 | 4.827% | 3.673% | 4.649% | 2.325% |
| 8 | 4.745% | 4.605% | 4.527% | 2.263% |
| Diff | 0.081% | -0.932% | 0.123% | 0.061% |
Here is the effect of the two rule changes thus far. So, the eight-deck game is still a little better.
- Player loses if both bust = -0.079%
- Blackjack pays 3-2 = 0.061%
- Total = -0.018%
Next, let's improve the player's strategy by using basic strategy with a hard total of 12 to 16, which is:
Player has 13 to 16 vs. dealer 2-6 = Stand
Player has 12 vs. dealer 4-6 = Stand
Otherwise, hit with hard 12 to 16.
Here is the effect of that strategy improvement.
- One deck = 3.703%
- Eight decks = 3.270%
- Difference = 0.433%
So this change in strategy is more helpful with a single deck. I assume this is because hitting with 12 to 16 is more dangerous in a single-deck game, because the 12 to 16 are likely composed of more small cards than large, resulting in hitting into a deck/shoe rich in high cards, thus more busting. In other words, the effect of removal again.
Here is where we are at now of the reason a single-deck game is better for the player.
- Player loses if both bust = -0.079%
- Blackjack pays 3-2 = 0.061%
- Strategic standing on 12 to 16 = 0.433%
- Total = 0.415%
Next, let's add proper doubling strategy. We will use the appropriate basic strategy for the given number of decks. Here is the value of adding doubling the player's game.
- One deck = 1.653%
- Eight decks = 1.380%
- Difference = 0.273%
Doubling is thus much more valuable in a single-deck game than a shoe. I figure it's because the player likely has two small cards when doubling, like a 6 and 5, resulting on doubling into a shoe/deck that is ten-card rich. Again, this effect of removal is stronger with one deck than eight.
Let's update our effect list:
- Player loses if both bust = -0.079%
- Blackjack pays 3-2 = 0.061%
- Strategic standing on 12 to 16 = 0.433%
- Strategic doubling = 0.273%
- Total = 0.689%
The only thing left to change (remember, we are not allowing surrender) is the splitting rule. Here is the effect of adding the appropriate splitting strategy:
- One deck = 0.544%
- Eight decks = 0.669%
- Difference = -0.125%
Interesting! Splitting is more effective in an 8-deck shoe. This should no be surprising as it is harder to form a pair in a single deck game than a shoe. Be be specific, the probabilitiy of getting any pair in two cards is 1/17 = 5.882%. In an eight deck shoe it is 7.470%. Not to get off topic, but it interesting how little splitting actually helps the player. So, there is more splitting and re-splitting going on in the shoe game.
Let's update our table again.
- Player loses if both bust = -0.079%
- Blackjack pays 3-2 = 0.061%
- Strategic standing on 12 to 16 = 0.433%
- Strategic doubling = 0.273%
- Strategic splitting = -0.125%
- Total = 0.563%
As a reminder, the difference from my blackjack house edge calculator matches the 0.563%. Yay!
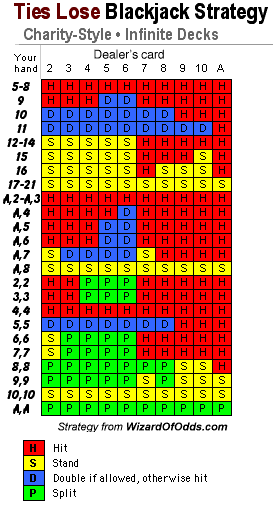
I love graphs, so here is one of the various effects I looked at.
Again, I plan to write this all up in a more formal way and will indclude more details. For now, I open it up to comments and questions. I've been working on this for about a week, so hope to get some feedback.
I would like to thank Don Schlesinger for his help and advice.
Again, I plan to write this all up in a more formal way and will indclude more details. For now, I open it up to comments and questions. I've been working on this for about a week, so hope to get some feedback.
Feedback, well lets use a story. Way back, like 2010, I was on a crew with a guy was no BJ dummy. His dad was a mill guy had to take a late in life dealer job. He knew all the basics. and was a good craps student the night I later taught him. The subject of continuous shufflers came up probably over breakfast or looking at oil leases in the courthouse.
Anyhow, I tried to explain how a CSM gives a slight player edge, having spent more time studying WoV and WoO than on any course I took in college. I kept telling him to read the article on it. A couple weeks of this and I sent him the link and he promised to read it.
At breakfast the next morning he said, 'Bro, that was way deeper than I imagined.' I had warned him it was, but he still just kind of took it on faith.
'Bro, that was way deeper than I imagined!'
I read this a few times but am still mostly just taking the math on faith. The only thing I could easily explain to a table at a party night is more chance to split because there are more matched cards with more decks. If you explained more of it like that it would be easier to understand.
Picture how I would have to explain it. At a table of people who have probably half of them played before, and most who are not 'math people.'
The first question I would get would be what is the difference with more decks since the ratio of cards is the same just more. A clump of small cards is as possible as a clump of large cards. At least that is how it appears.
Maybe show how things differ going form 1 to 2 decks, then build on that. Then look at some random hands. Say player 10, 12, and 16. What are my probabilities of hitting 12 on 1 deck vs 2. Ditto 16. Think how many ways to bust a 16 with one deck then 2. How many ways to improve on 1 deck then 2. If I had something that simple, even if for just say 12 and 16 for 1 vs 2 decks I could explain it to people at a table.
IOW, can you show it with cards and not decimals?
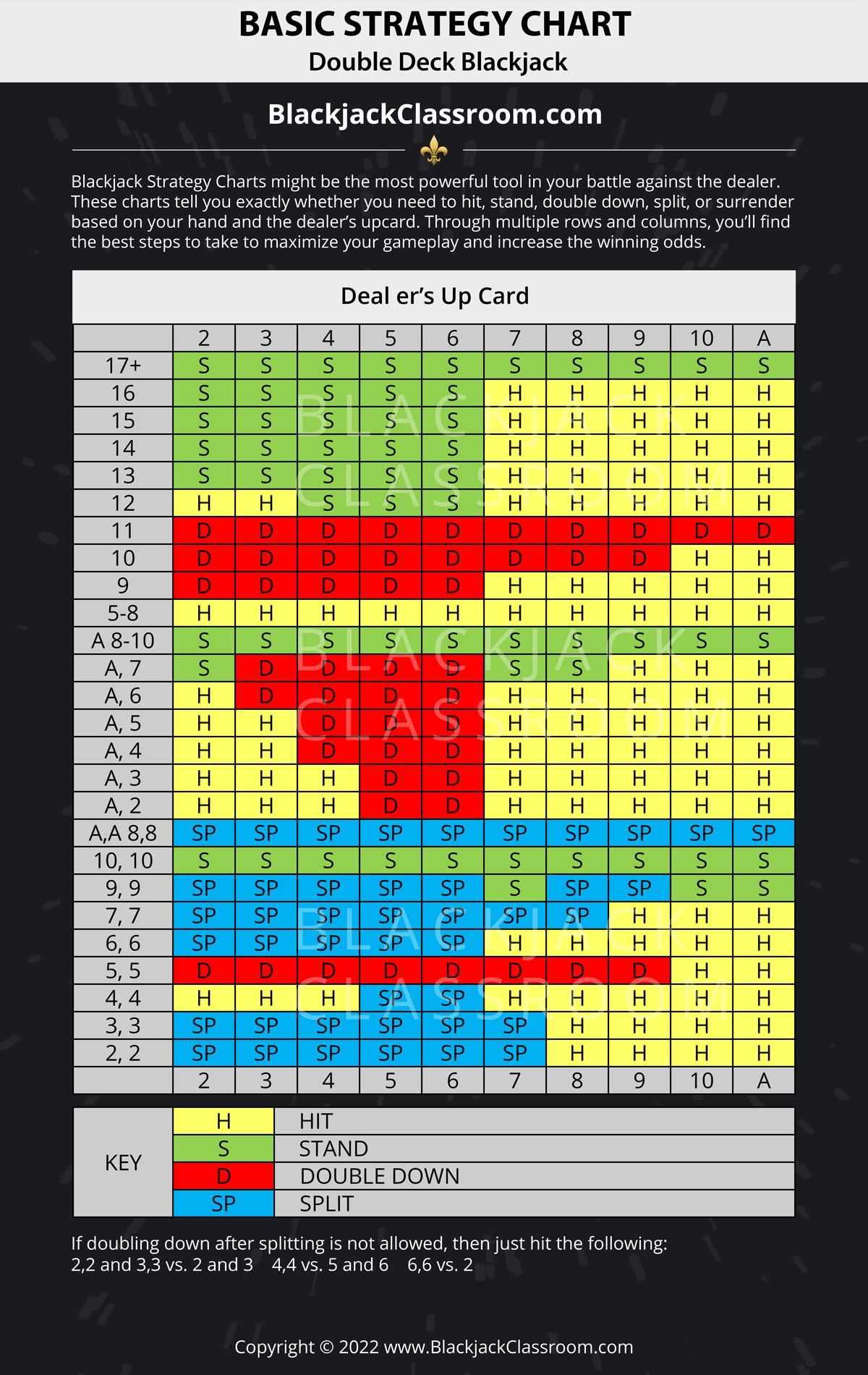 Just a thought.
Just a thought.Administrator
Second, it's always been my policy to teach to near the top. If you put everybody on the bell curve, I try to aim at the person about one standard deviation above average (meaning 84% are less intelligent and 16% are more). There are already plenty of advanced books for those 2 or 3 standard deviations above the norm and plenty of sources for those who need things simplified as much as possible. I aim for those in between.
The topic at hand is complicated and difficult to dumb down. Other sources on this question tend to emphasize that blackjacks are more likely the fewer the decks and the player gets that extra half unit on a winning blackjack. That is pretty easy to explain, but it accounts for about 11% of the total effect of the number of decks only.
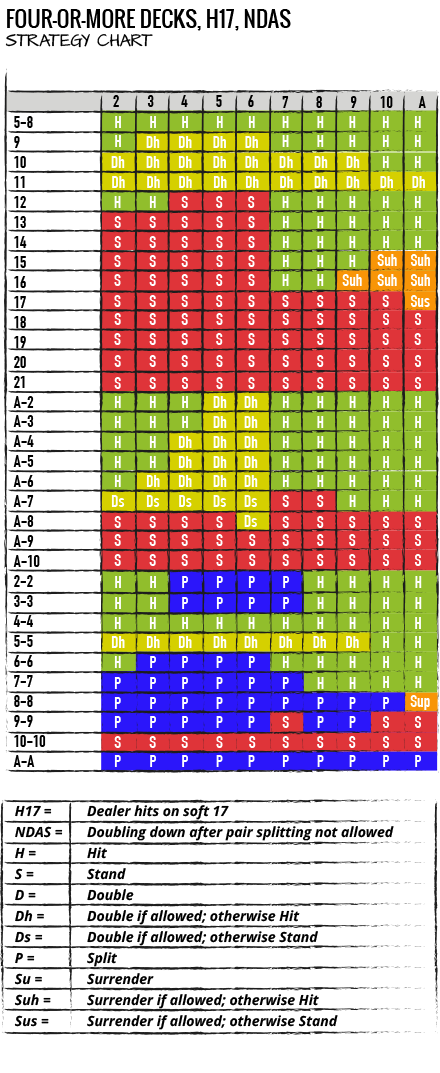
Nevertheless, I appreciate the suggestion.
I will read Wizards post several more times in the coming days and weeks, and it is possible some things might click and make sense as time goes on, but the majority of it will remain a foreign language to me. :/
Same thing happened with Don's BJA3. First time I read it....foreign language. Understood almost none of the math, But I applied many of the conclusions. BJA3 happens to be my Bible. I have re-read that 100 times over the years and frequently reference different things, and every once in a while something 'clicks' and I say oh yeah that makes sense, but even still most of the math is foreign to me. I am probably the stupidest, most math challenged person on this forum or in the blackjack community. I'm ok with that.
Kind of like a car engine. I drive a vehicle, but if you tell me that the spark tube (The Office mafia episode reference) is bad and needs to be replaced, you are talking a foreign language.
so in another thread Wizard suggested I take a look at this thread. And so I have. I have to say none of the conclusions surprised me. I knew most of that. But the math to get there....that is another matter. Despite that I play blackjack for a living, I am not a math guy nor understand many of the math and formulas behind things. I take what other, much smarter people than me conclude and apply it. People like Wizard, Don Schlesinger, Doghand and many others.
I will read Wizards post several more times in the coming days and weeks, and it is possible some things might click and make sense as time goes on, but the majority of it will remain a foreign language to me. :/
Same thing happened with Don's BJA3. First time I read it....foreign language. Understood almost none of the math, But I applied many of the conclusions. BJA3 happens to be my Bible. I have re-read that 100 times over the years and frequently reference different things, and every once in a while something 'clicks' and I say oh yeah that makes sense, but even still most of the math is foreign to me. I am probably the stupidest, most math challenged person on this forum or in the blackjack community. I'm ok with that.
Kind of like a car engine. I drive a vehicle, but if you tell me that the spark tube (The Office mafia episode reference) is bad and needs to be replaced, you are talking a foreign language.
What about Griffin’s Theory of Blackjack? Fuggedaboudit!
What about Griffin’s Theory of Blackjack? Fuggedaboudit!
I'm still waiting on the English language version to be published.
Maybe it's just me, but I never quite get over how when you are actually playing you get no sense that any of this is in play. For me in fact I get no sense whatsoever while playing BJ that the real advantage the dealer has is the rule about who busts first. Instead, I might get irritated by how many BJs he gets, how he can draw a bunch of small cards when he needs them, or how often he deals me a 12-16 hand while he so often gets great starting cards. Yeah, 'seemingly' should have been inserted in all the above. The sense I get, though, it doesn't seem like 'seemingly'
Blackjack Number Chart
Busting rule? Not a hint that is a problem!In Craps, when playing the darkside, the idea that a 12 rolled on the comeout is a push instead of a win is the real killer is about as hidden an effect as you can possibly experience. I in fact have experienced some awful sessions where a 12 was rolled on the comeout maybe only once the entire time. It is so counter-intuitive to grasp the real reasons the house has an edge in these things!
Administrator
It is so counter-intuitive to grasp the real reasons the house has an edge in these things!
Number Of Decks In Blackjack Tournaments
That is one of the essences of a well-designed casino game -- the player doesn't see where the house has the advantage. In blackjack and most poker games it is a player positional disadvantage. The idea to remove the 10's in Spanish 21 was also a good one.I get asked from time to time by the other side about how to significantly increase the house edge, like by 1%, in blackjack without changing the 3-2 payoff, mandating a side bet, charging a commission on wins, nor change the deck composition in a way the average player won't notice. Nothing good has ever come to mind.
That is one of the essences of a well-designed casino game -- the player doesn't see where the house has the advantage. In blackjack and most poker games it is a player positional disadvantage. The idea to remove the 10's in Spanish 21 was also a good one.
I get asked from time to time by the other side about how to significantly increase the house edge, like by 1%, in blackjack without changing the 3-2 payoff, mandating a side bet, charging a commission on wins, nor change the deck composition in a way the average player won't notice. Nothing good has ever come to mind.
The most popular blackjack game nowadays is played with 8 decks of cards. Though it may seem impossible to count cards here, below you can see that the chances are still high that you will improve your odds and the graphics shows you the frequencies the count will occur.
% of times occurred in the games
Count
Number Of Decks In Blackjack Card Game
Though the chances of having a very high or very low count are extremely low, you can still use the count to your advantage. Have a look at the table below to see the advantages.
| Count | % | Prob. this or more |
| -49 | 0,00000003% | 100,00000000% |
| -48 | 0,00000006% | 99,99999997% |
| -47 | 0,00000021% | 99,99999991% |
| -46 | 0,00000027% | 99,99999970% |
| -45 | 0,00000033% | 99,99999943% |
| -44 | 0,00000021% | 99,99999910% |
| -43 | 0,00000021% | 99,99999889% |
| -42 | 0,00000042% | 99,99999868% |
| -41 | 0,00000057% | 99,99999826% |
| -40 | 0,00000129% | 99,99999769% |
| -39 | 0,00000267% | 99,99999640% |
| -38 | 0,00000577% | 99,99999372% |
| -37 | 0,00001192% | 99,99998796% |
| -36 | 0,00002135% | 99,99997603% |
| -35 | 0,00003997% | 99,99995468% |
| -34 | 0,00006874% | 99,99991471% |
| -33 | 0,00012276% | 99,99984597% |
| -32 | 0,00020336% | 99,99972321% |
| -31 | 0,00034258% | 99,99951985% |
| -30 | 0,00056772% | 99,99917727% |
| -29 | 0,00094862% | 99,99860955% |
| -28 | 0,00148646% | 99,99766093% |
| -27 | 0,00242661% | 99,99617447% |
| -26 | 0,00378132% | 99,99374787% |
| -25 | 0,00570811% | 99,98996654% |
| -24 | 0,00861279% | 99,98425844% |
| -23 | 0,01276072% | 99,97564564% |
| -22 | 0,01881787% | 99,96288492% |
| -21 | 0,02687454% | 99,94406705% |
| -20 | 0,03836799% | 99,91719252% |
| -19 | 0,05366363% | 99,87882453% |
| -18 | 0,07276165% | 99,82516090% |
| -17 | 0,10027183% | 99,75239925% |
| -16 | 0,13420432% | 99,65212741% |
| -15 | 0,17767643% | 99,5179% |
| -14 | 0,23517096% | 99,3402% |
| -13 | 0,30721438% | 99,1051% |
| -12 | 0,39385820% | 98,7979% |
| -11 | 0,49583814% | 98,4040% |
| -10 | 0,65706943% | 97,9% |
| -9 | 0,81199775% | 97,3% |
| -8 | 1,03675913% | 96,4% |
| -7 | 1,37508469% | 95,4% |
| -6 | 1,80959369% | 94,0% |
| -5 | 2,44941697% | 92,2% |
| -4 | 3,44408967% | 89,8% |
| -3 | 5,10863060% | 86,3% |
| -2 | 8,06068240% | 81,2% |
| -1 | 13,84397685% | 73,2% |
| 0 | 18,70279601% | 59,3% |
| 1 | 13,82825814% | 40,6% |
| 2 | 8,04279303% | 26,8% |
| 3 | 5,09367991% | 18,7% |
| 4 | 3,43603877% | 13,6% |
| 5 | 2,44108946% | 10,2% |
| 6 | 1,80182387% | 7,8% |
| 7 | 1,36877544% | 6,0% |
| 8 | 1,03208321% | 4,6% |
| 9 | 0,80882601% | 3,6% |
| 10 | 0,65469463% | 2,8% |
| 11 | 0,49454697% | 2,1000% |
| 12 | 0,39331168% | 1,6055% |
| 13 | 0,30716922% | 1,2121% |
| 14 | 0,23571526% | 0,9050% |
| 15 | 0,17839949% | 0,6693% |
| 16 | 0,13512375% | 0,49086273% |
| 17 | 0,10143784% | 0,35573898% |
| 18 | 0,07382084% | 0,25430114% |
| 19 | 0,05474817% | 0,18048030% |
| 20 | 0,03928402% | 0,12573213% |
| 21 | 0,02759649% | 0,08644811% |
| 22 | 0,01944327% | 0,05885162% |
| 23 | 0,01332745% | 0,03940835% |
| 24 | 0,00906333% | 0,02608090% |
| 25 | 0,00602366% | 0,01701757% |
| 26 | 0,00406264% | 0,01099390% |
| 27 | 0,00264117% | 0,00693126% |
| 28 | 0,00163309% | 0,00429009% |
| 29 | 0,00105093% | 0,00265700% |
| 30 | 0,00063724% | 0,00160606% |
| 31 | 0,00039910% | 0,00096883% |
| 32 | 0,00024387% | 0,00056973% |
| 33 | 0,00014429% | 0,00032585% |
| 34 | 0,00008012% | 0,00018156% |
| 35 | 0,00004381% | 0,00010144% |
| 36 | 0,00002438% | 0,00005763% |
| 37 | 0,00001429% | 0,00003324% |
| 38 | 0,00000802% | 0,00001895% |
| 39 | 0,00000435% | 0,00001093% |
| 40 | 0,00000312% | 0,00000658% |
| 41 | 0,00000189% | 0,00000345% |
| 42 | 0,00000087% | 0,00000156% |
| 43 | 0,00000045% | 0,00000069% |
| 44 | 0,00000012% | 0,00000024% |
| 45 | 0,00000009% | 0,00000012% |
| 46 | 0,00000003% | 0,00000003% |